Normalized frequency (digital signal processing)
In digital signal processing, the normalized frequency of a periodic signal is its frequency expressed in units of cycles (or radians) per sample, rather than in the usual SI units of hertz (cycles per second). The cycles-per-sample frequency is computed by dividing the cycles-per-second frequency by the sampling rate (samples per second); symbolically, the "/second" (per second) units cancel:
- (cycles/second) / (samples/second) = cycles/sample.
Contents |
Applications
The abstract reason for using normalized frequency is that, from the point of view of signal processing, a second is an arbitrary unit of time, while the sampling interval is a meaningful quantity (formally, a characteristic unit for the system): the frequency of a signal with respect to 1 second does not tell you about the behavior of the signal, but the frequency of a signal with respect to the sampling interval tells you the effect of sampling on the signal, via the sampling theorem. Stated alternatively, this process is called "normalization", and the sampling frequency is a normalizing constant.
In filter design, a given design can be used at different sample-rates, resulting in different frequency responses. Normalization produces a distribution that is independent of the sample rate, and thus one plot is sufficient for all possible sample rates.
Alternative normalizations
The reference value is usually the sampling frequency, denoted 
in samples per second, because the frequency spectrum of a sampled signal (with real or complex values) is periodic with period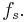 
When the actual frequency
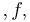has units of hertz (SI units), the normalized frequencies, also denoted by 
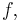have units of cycles per sample, and the periodicity of the normalized spectrum is 1.
Alternatively, if the actual frequency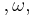
is written with units of radians per second (angular frequency), the normalized frequencies have units of radians per sample, and the periodicity of the distribution is 2π.
If a sampled waveform is real-valued, such as a typical filter impulse response, the periodicity of the frequency distribution is still 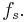But due to symmetry, it is completely defined by the content within a span of just 
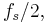half the sampling frequency – the Nyquist frequency. Accordingly, some filter design procedures/applications use that as the normalization reference (and the resulting units are half-cycles per sample).
Example
The following table shows examples of normalized frequencies for a 1 kHz signal, a sample rate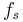 
= 44.1 kHz, and these 3 different choices of normalization constant
| Type | Computation | Value |
| Radians/sample | 2 π 1000 / 44100 | 0.1425 |
| cycles/sample (w.r.t. fs, sampling frequency) |
1000 / 44100 | 0.02268 |
| half-cycles/sample (w.r.t. fs/2, Nyquist frequency) |
1000 / 22050 | 0.04535 |